Divergence-Free Shape Correspondence by Deformation
Authored by Marvin Eisenberger, Zorah Lähner, Daniel Cremers
Published in Symposium on Geometry Processing (SGP) 2019
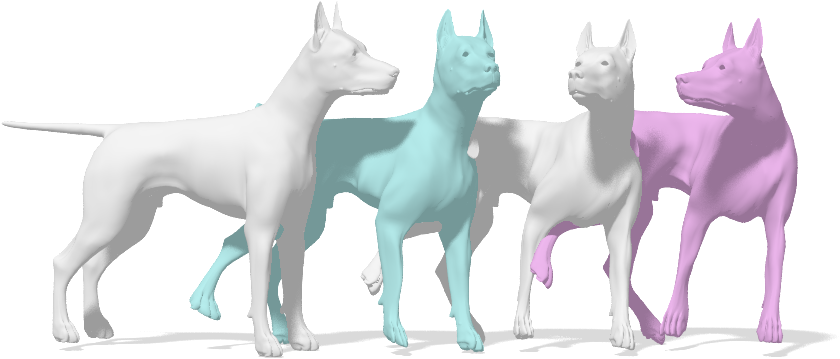
Abstract
We propose the first algorithm for non-rigid 2D-to-3D shape matching, where the input is a 2D query shape as well as a 3D target shape and the output is a continuous matching curve represented as a closed contour on the 3D shape. We cast the problem as finding the shortest circular path on the product 3-manifold of the two shapes. We prove that the optimal matching can be computed in polynomial time with a (worst-case) complexity of O(mn^2 log(n)), where m and n denote the number of vertices on the 2D and the 3D shape respectively. Quantitative evaluation confirms that the method provides excellent results for sketch-based deformable 3D shape retrieval.
Resources
Bibtex
@inproceedings{ eisenberger2019divfree,
author = { Marvin Eisenberger and Zorah Lähner and Daniel Cremers },
title = { Divergence-Free Shape Correspondence by Deformation },
booktitle = { Symposium on Geometry Processing (SGP) },
year = { 2019 },
}